When exposed to an AC electric field, a piezoelectric ceramic element changes dimensions cyclically, at the cycling frequency of the field. The frequency at which the ceramic element vibrates most readily, and most efficiently converts the electrical energy input into mechanical energy, is the resonance frequency.

The pattern of an element’s responses is depicted in Figure 1.8. As the frequency of cycling is increased, the element’s oscillations first approach a frequency at which impedance is minimum (maximum admittance). This minimum impedance frequency, fm , approximates the series resonance frequency, fs , the frequency at which impedance in an electrical circuit describing the element is zero, if resistance caused by mechanical losses is ignored. The minimum impedance frequency also is the resonance frequency, fr. The composition of the ceramic material and the shape and volume of the element determine the resonance frequency — generally, a thicker element has a lower resonance frequency than a thinner element of the same shape.

As the cycling frequency is further increased, impedance increases to a maximum (minimum admittance). The maximum impedance frequency, fn , approximates the parallel resonance frequency, fp , the frequency at which parallel resistance in the equivalent electrical circuit is infinite if resistance caused by mechanical losses is ignored. The maximum impedance frequency also is the anti-resonance frequency, fa. Maximum response from the element will be at a point between fm and fn.
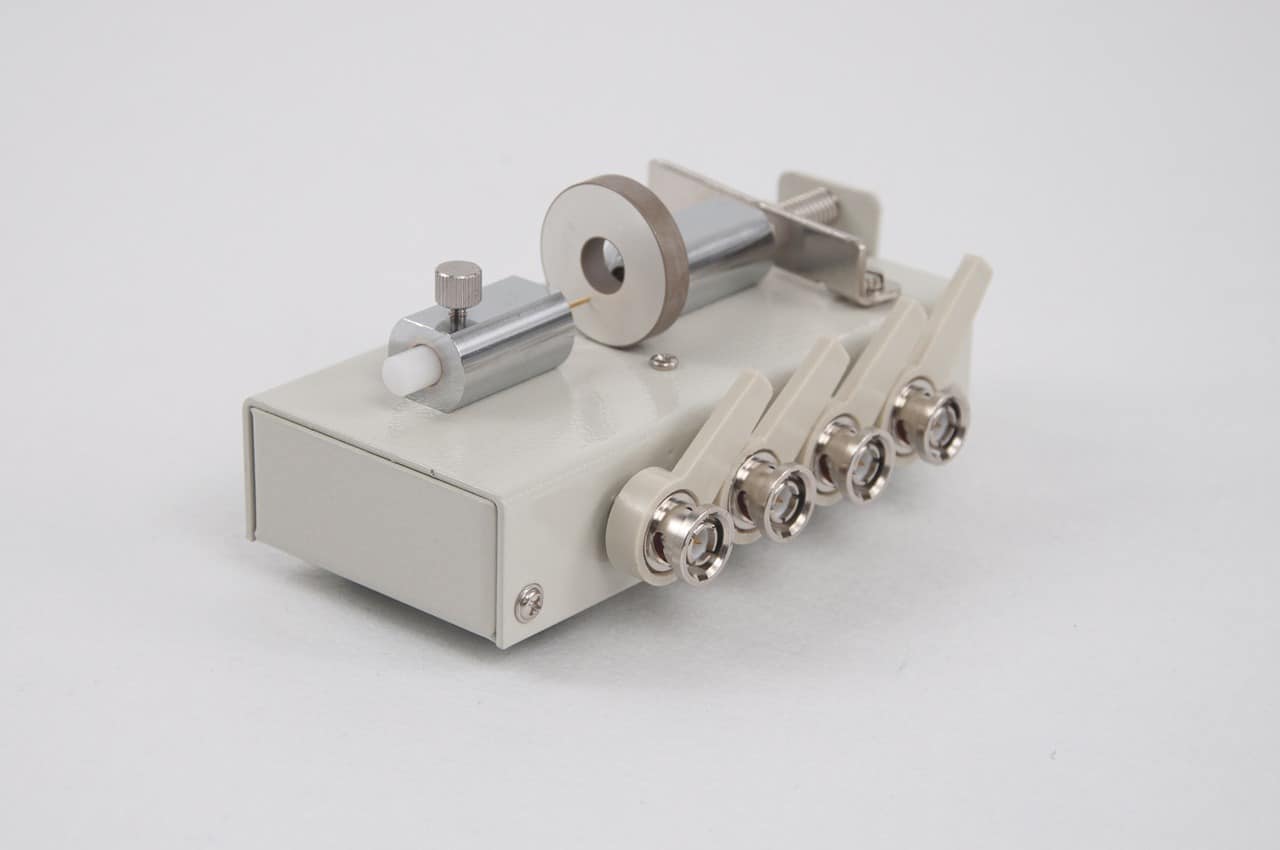
Values for minimum impedance frequency, fm , and maximum impedance frequency, fn , can be determined by measurement. Figure 1.10 shows a system designed to ascertain these values, and summarizes the procedure.

Impedance & Resonance Frequency Impedance as a Function of Cycling Frequency
A ceramic element’s oscillations first approach the minimum impedance frequency (fm ) / resonance frequency (fr ), at which the element vibrates most readily, and most efficiently converts electrical energy into mechanical energy. As cycling frequency is further increased, impedance increases to the maximum impedance frequency (fn ) / anti-resonance frequency (fa ).

Procedure:
Set the switch to A.
Place the ceramic element into position.
Adjust the frequency generator to give a maximum voltage value on the voltmeter. This value is the resonance frequency.
Set the switch to B.
Adjust R4 to give a voltage value on the voltmeter equal to the value in step 3. This value is the impedance resonance (Zr).
Set the switch to A.
Adjust the frequency generator to give a minimum voltage value on the voltmeter. This value is the antiresonance frequency.

fm and fn can be used to calculate the electromechanical coupling factor, k. k depends on the mode of vibration and the shape of the ceramic element. The relationships between k and fm and fn for a ceramic plate, a disc (surface dimensions large, relative to thickness), or a rod are:

Coupling Factor for Plates / k31*

(π/2)(fn/fm)tan[(π/2)(fn-fm)/fm)]

K312=——————————————————-

1+(π/2)(fn/fm)tan[(π/2)(fn-fm)/fm)]

* electric field parallel to direction of polarization,
induced strain perpendicular to direction of polarization
Coupling Factor for Discs / kp**

Kp ≈√[(2.51(fn-fm)/fn-(fn-fm)/fn2]

** electric field parallel to direction of polarization,
induced strain in same direction
Coupling Factor for Rods / k33***

K332=(π/2)(fn/fm)tan[(π/2)(fn-fm)/fn]

*** electric field parallel to direction of polarization,
induced strain in same direction